مكانيك محيط هاي پيوسته

تحليل سينماتيكي و رفتار مكانيكي مواد مدل شده بر اساس فرضيه محيط هاي پيوسته را مكانيك محيط هاي پيوسته (Continuum Mechanics) مي نامند. در درس مكانيك محيط هاي پيوسته، علاوه بر فرضيه بنيادين پيوستگي مواد، دو محدوديت ديگر را روي اجسام در نظر مي گيريم:

• اولا الزام مي كنيم كه مواد همگن باشند، يعني خواص مكانيكي يكسان در تمام موقعيت ها داشته باشند.
• ثانيا بطور كلي موادي را در نظر مي گيريم كه همسانگرد هستند، يعني در يك نقطه داراي خواص فيزيكي يكسان در هر جهت مي باشند...

مجموعه آموزشي مكانيك محيط هاي پيوسته براي مهندسين توماس ميس، يكي از مجموعه كتاب هاي مرجع و كاربردي در زمينه مكانيك محيط هاي پيوسته است كه در اكثر دانشگاه هاي ايران تدريس مي گردد. در اين مجموعه آموزشي شما قادر خواهيد بود اولا نسخه اصلي كتاب به زبان لاتين (Introduction to Continuum Mechanics)، ثانيا نسخه ترجمه شده اين كتاب به زبان فارسي (مترجم دكتر عباس راستگو)، ثالثا تشريح كامل مسائل اين كتاب را بطور كامل دانلود نماييد.

كتاب مكانيك محيط هاي پيوسته براي مهندسين،
مشتمل بر 440 صفحه، در 9 فصل، با فرمت PDF، به زبان فارسي، همراه با تصاوير به ترتيب زير گردآوري شده است:

فصل 1: نظريه محيط هاي پيوسته

• مفهوم محيط هاي پيوسته
• مكانيك محيط هاي پيوسته

فصل 2: رياضيات ضروري

• عددها، بردارها و تانسورهاي قائم
• تانسور جبري در نمادگذاري نمادين، قرارداد جمع
• نمادگذاري شاخصي
• ماتريس ها و دترمينان ها
• انتقال ماتريس هاي قائم
• مقادير اصلي و جهت هاي اصلي تانسورهاي متقارن مرتبه دوم
• ميدان هاي تانسوري، حسابان تانسورها
• نظريه هاي انتگرالي گوس و استوكس
• مسائل

فصل 3: اصول تنش

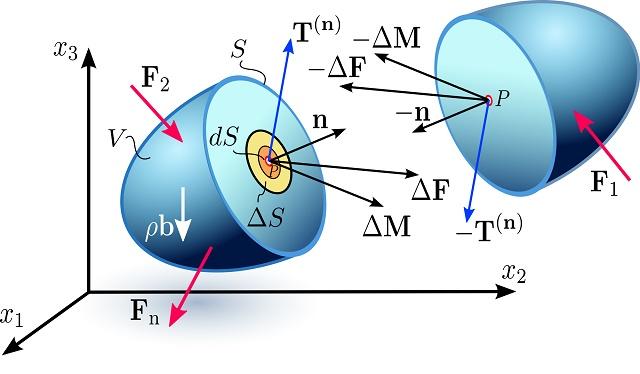
• نيروهاي حجمي و سطحي، چگالي جرم
• اصل تنش كوشي
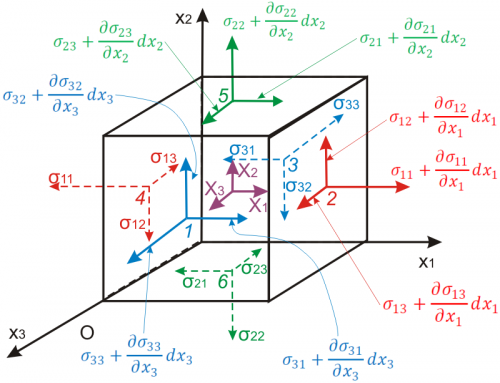
• تانسور تنش
• تعادل نيرو و ممان، تقارن در تانسور تنش
• قوانين انتقال تنش
• تنش هاي اصلي، جهت هاي تنش اصلي
• مقادير تنش حداقل و حداكثر
• دايره هاي مور براي تنش
• تنش صفحه اي
• بيان تنش كروي و بيان تنش انحراف
• تنش برشي هشت وجهي
• مسائل

فصل 4: سينماتيك تغير شكل و حركت

• ذرات، پيكربندي ها، تغير شكل و حركت
• مختصات مادي و فضايي
• توصيف هاي اولري و لاگرانژي
• ميدان تغير مكان
• مشتق مادي
• گراديان تغير شكل، تانسورهاي كرنش محدود
• نظريه تغير شكل بسيار كوچك
• نسبت هاي كشيدگي
• تانسور چرخش، تانسورهاي كشيدگي
• گراديان سرعت، نرخ تغير شكل، گردابي
• مشتق مادي اجزاء خطي، مساحت ها، حجم ها
• مسائل

فصل 5: قوانين و معادلات بنيادين

• قوانين تعادل، معادلات ميدان، معادلات اساسي
• مشتقات مادي انتگرال هاي خط، سطح و حجم
• اصل بقاي جرم، معادله پيوستگي
• اصل مومنتوم خطي، معادلات حركت
• تانسورهاي تنش پيلا كرشوف، معادلات لاگرانژ حركت
• اصل ممان اندازه حركت (اندازه حركت زاويه اي)
• اصل بقاي انرژي، معادله انرژي
• آنتروپي و معادله كلازيوس دوهام
• محدوديت هايي بر مواد الاستيك بوسيله قانون دوم ترموديناميك
• تغير ناپذيري
• محدوديت هاي اعمال شده بر معادله هاي اساسي بواسطه تغير ناپذيري
• معادلات اساسي
• مسائل

فصل 6: الاستيسيته خطي

• الاستيسيته، قانون هوك، انرژي كرنشي
• قانون هوك براي مواد همسانگرد، ثابت هاي الاستيك
• تقارن الاستيك، قانون هوك براي مواد غير همسانگرد
• الاستو الاستيك و الاستو ديناميك همسانگرد، اصل جمع آثار
• الاستيسيته صفحه اي
• تابع تنش ايري
• پيچش
• الاستيسيته سه بعدي
• مسائل

فصل 7: شاره هاي كلاسيك

• تانسور تنش چسبنده، شاره هاي استوكسي و نيوتني
• معادلات اصلي جريان چسبنده، معادلات ناوير استوكس
• شاره هاي ويژه
• جريان يكنواخت، جريان غير چرخشي، جريان پتانسيل
• معادله برنولي، قضيه كلوين
• مسائل

فصل 8: الاستيسيته غير خطي

• روش مولگولي براي الاستيسيته لاستيك
• تئوري انرژي كرنشي براي الاستيسيته غير خطي
• حتالت هاي خاص انرزي كرنشي
• حل دقيق براي يك ماده تراكم ناپذير و نئو هوكين
• مسائل

فصل 9: ويسكو الاستيسيته خطي

• مقدمه
• معادلات اساسي ويسكو الاستيك در شكل اپراتور ديفرانسيل خطي
• تئوري يك بعدي، مدل هاي مكانيكي
• خزش و وارفتگي
• اصل برهم نهي، انتگرال هاي توارثي
• بارگذاري هاي هارمونيك، مدول مختلط، مطلوبيت مختلط
• مسائل سه بعدي، اصل تطابق
• مسائل

كتاب مكانيك محيط هاي پيوسته براي مهندسين (Continuum Mechanics for Engineers)،
مشتمل بر 400 صفحه، در 9 فصل، با فرمت PDF، به زبان انگليسي، همراه با مسائل به ترتيب زير گردآوري شده است:

• Chapter 1: Continuum Theory
• Chapter 2: Essential Mathematics
• Chapter 3: Stress Principles
• Chapter 4: Kinematics of Deformation and Motion
• Chapter 5: Fundamental Laws and Equations
• Chapter 6: Linear Elasticity
• Chapter 7: Classical Fluids
• Chapter 8: Nonlinear Elasticity
• Chapter 9: Linear Viscoelasticity

كتاب تشريح كامل مسائل مكانيك محيط هاي پيوسته براي مهندسين،
مشتمل بر 220 صفحه، با فرمت PDF، به زبان فارسي، كليه مسائل كتاب بالا را بطور كامل تشريح نموده است و به ترتيب زير گردآوري شده است:

• رياضيات ضروري
• اصول تنش
• سينماتيك تغير شكل و حركت
• قوانين و معادلات بنيادين
• الاستيسيته خطي
• شاره هاي كلاسيك

جهت دانلود مجموعه آموزشي مكانيك محيط هاي پيوسته به همراه تشريح كامل مسائل، برلينك زير كليك نماييد.

مجموعه آموزشي مكانيك محيط هاي پيوسته به همراه تشريح كامل مسائل

كتاب تشريح كامل مسائل مكانيك محيط هاي پيوسته براي مهندسان، مشتمل بر 220 صفحه، با فرمت PDF، به زبان فارسي، زير نظر دكتر عباس راستگو، به ترتيب زير گردآوري شده است:

• رياضيات ضروري
• اصول تنش
• سينماتيك تغير شكل و حركت
• قوانين و معادلات بنيادين
• الاستيسيته خطي
• شاره هاي كلاسيك

در اين كتاب شما با نحوه حل دقيق مسائل مكانيك محيط هاي پيوسته (Continuum Mechanics) با زبان گويا آشنا شده و با تمرين و ممارست مي توانيد به راحتي هر مسئله مكانيك محيط هاي پيوسته را حل نماييد.

جهت دانلود كتاب تشريح كامل مسائل مكانيك محيط هاي پيوسته براي مهندسان، برلينك زير كليك نماييد.

تشريح كامل مسائل مكانيك محيط هاي پيوسته براي مهندسان

مكانيك محيط هاي پيوسته، يك شاخه وسيع از مكانيك محسوب مي شود. هدف اين شاخه علمي، فراگيري اين واقعيت است كه چگونه مسائل مربوط به حركت اجسام شكل پذير (جامد، مايع و گاز) صورت بندي و سئوالات و ايده هاي كلي و حتي مبهم به عبارات دقيق رياضي بدل شوند. در زمينه مكانيك محيط هاي پيوسته، كتب متعددي تدوين شده است. كتاب مقدمه اي بر مكانيك محيط هاي پيوسته (مايكل لي، ديويد رابين، ارهارد كرمپل) به دو دليل زير به شما علاقمندان پيشنهاد مي گردد:

نخست اينكه سادگي در بيان مطالب، تقسيم بندي مناسب موضوعات، ارائه مثال هاي متعدد براي تفهيم مطلب و گردآوري مسائل زياد در انتهاي هر فصل، كتاب را براي كلاس درس كاملا مناسب نموده است. دوم اينكه با توجه به معرفي تانسورهاي مرتبه دو از طريق تشريح تبديلات خطي، به فهم مبادي رياضي متن كه يكي از پيچيدگي هاي مكانيك محيط هاي پيوسته محسوب مي شود، سادگي و سهولت خاصي بخشيده است...

كتاب مقدمه اي بر مكانيك محيط هاي پيوسته، يكي از كتاب هاي مرجع و كاربردي در زمينه مكانيك محيط هاي پيوسته است كه در اكثر دانشگاه هاي ايران تدريس مي گردد. در اين مجموعه آموزشي شما قادر خواهيد بود اولا نسخه اصلي كتاب به زبان لاتين (Introduction to Continuum Mechanics)، ثانيا نسخه ترجمه شده اين كتاب به زبان فارسي (مترجم دكتر غلامحسين رحيمي)، ثالثا تشريح كامل مسائل اين كتاب را بطور كامل دانلود نماييد.

اين كتاب مشتمل بر 375 صفحه، در 8 فصل، با فرمت PDF، به زبان فارسي، همراه با مسائل به ترتيب زير گردآوري شده است:

فصل 1: مقدمه

• نظريه محيط پيوسته
• محتواي مكانيك محيط هاي پيوسته

فصل 2: نمادگذاري شاخصي و تانسورها

• قرارداد جمع، شاخص هاي كاذب
• شاخص هاي آزاد
• دلتاي كرانكر
• نماد جايگشت
• عمليات با نمادگذاري شاخصي
• تانسور، يك تبديل خطي
• مولفه هاي يك تانسور
• جمع تانسور
• حاصلضرب دياديك
• ضرب دو تانسور
• تانسور واحد
• برگردان يك تانسور
• تانسور متعامد
• قوانين تبديل براي مولفه هاي دكارتي تانسورها و بردارها
• تانسورهاي متقارن و پادمتقارن
• بردار دوگان يك تانسور پادمتقارن
• مقادير ويژه و بردارهاي ويژه يك تانسور
• مقادير اصلي و جهات اصلي تانسورهاي متقارن حقيقي
• ماتريس يك تانسور نسبت به جهات اصلي
• پاياهاي عددي يك تانسور
• توابع با ارزش تانسوري يك عددي
• ميدان عددي، گراديان يك تابع عددي
• ميدان برداري، گراديان يك تابع برداري
• اثر يك تانسور مرتبه دو
• ديورژانس يك ميدان برداري و ديورژانس يك ميدان تانسوري
• كرل يك ميدان برداري
• مختصات قطبي
• مسائل

فصل 3: سينماتيك محيط هاي پيوسته

• توصيف حركت يك محيط پيوسته
• توصيف مادي و توصيف فضايي
• مشتق مادي
• يافتن شتاب يك ذره از يك ميدان سرعت داده شده
• تغير شكل
• كرنش اصلي
• اتساع
• نرخ تغير شكل
• معادله بقاي جرم
• شرايط سازگاري براي مولفه هاي بي نهايت كوچك كرنش
• شرايط سازگاري براي مولفه هاي نرخ تغيير شكل
• مسائل

فصل 4: تنش

• بردار تنش
• تانسور تنش
• مولفه هاي تانسور تنش
• تقارن تانسور تنش، اصل ممان اندازه حركت
• تنش هاي اصلي
• تنش برشي حداكثر
• معادلات حركت، اصل اندازه حركت خطي
• شرط مرزي براي تانسور تنش
• مسائل

فصل 5: جامد الاستيك خطي

• خواص مكانيكي
• جامد الاستيك خطي
• جامد الاستيك همسانگرد خطي
• مدول يانگ، ضريب پواسون، مدول برشي و مدول حجمي
• معادلات نظريه بي نهايت كوچك الاستيسيته
• اصل جمع آثار
• مثال هاي از الاستو ديناميك
• موج غير چرخشي مسطح
• موج هم حجم مسطح
• انعكاس امواج الاستيك مسطح
• ارتعاش يك ورق بي نهايت
• مثال هايي از الاستو استاتيك
• كشش ساده
• پيچش يك استوانه مدور
• پيچش يك استوانه غير مدور
• خمش خالص يك تير
• كرنش مسطح
• مسائل

فصل 6: سيال چسبنده نيوتني

• سيالات
• سيالات تراكم پذير و تراكم ناپذير
• معادلات هيدرو استاتيك
• سيال نيوتني
• تفسير لاندا و مو
• سيال نيوتني تراكم ناپذير
• شرايط مرزي
• خط جريان، خط مسير، جريان پايدار و ناپايدار، جريان لايه لايه و مغشوش
• مثال هاي از جريان هاي لايه لايه يك سيال نيوتني تراكم ناپذير
• جريان كوئت مسطح
• جريان پوسله مسطح
• جريان هاگن پوسوله
• جريان كوئت مسطج از دو لايه سيال تراكم ناپذير
• جريان كوئت
• جريان نزديك يك ورق مرتعش
• نرخ كار انجام شده روي يك ذره
• نرخ سيلان حرارت به داخل يك المان
• معادله انرژي
• بردار چرخش
• جريان غير چرخشي
• جريان غير چرخشي يك سيال تراكم ناپذير غير چسبنده با چگالي همگن
• جريان هاي غير چرخشي به عنوان حلي براي معادله ناوير استوكس
• معادله انتقال چرخش براي سيال چسبنده تراكم ناپذير با چگالي ثابت
• مفهوم لايه مرزي
• سيال نيوتني تراكم پذير
• معادله انرزي بر حسب آنتالپي
• موج صوتي
• جريان هاي باروتروپيك و غير چرخشي سيال تراكم پذير غير چسبنده
• جريان يك بعدي يك سيال تراكم پذير
• مسائل

فصل 7: فرمول بندي انتگرالي اصول عمومي

• قضيه گرين
• قضيه ديورژانس
• انتگرال روي يك حجم كنترل و انتگرال روي يك سطح مادي
• اصل بقاي جرم
• اصل مقدار حركت خطي
• پيرامون حجم كنترل متحرك
• اصل ممان اندازه حركت
• اصل بقاي انرژي
• مسائل

فصل 8: سيال ساده تراكم ناپذير

• هيات جاري، به عنوان هيات مرجع
• تانسور تغيير شكل نسبي
• تانسور سابقه تغيير شكل، تانسورهاي رولين اريكسن
• سيال ساده تراكم ناپذير
• سيال رولين اريكسن
• جريان هاي ويسكومتر يك سيال ساده تراكم ناپذير
• تنش ها در جريان ويسكومتر يك سيال ساده تراكم ناپذير
• جريان هاي برشي ساده
• جريان در كنال
• مسائل

كتاب مقدمه اي بر مكانيك محيط هاي پيوسته (Introduction to Continuum Mechanics)، مشتمل بر 536 صفحه، در 8 فصل، با فرمت PDF، به زبان انگليسي، همراه با مسائل به ترتيب زير گردآوري شده است:

• Chapter 1: Introduction
• Chapter 2: Tensors
• Chapter 3: Kinematics of a Continuum
• Chapter 4: Stress and Integral Formulations of General Principles
• Chapter 5: The Elastic Solid
• Chapter 6: Newtonian Viscous Fluid
• Chapter 7: The Reynolds Transport Theorem and Applications
• Chapter 8: Non-Newtonian Fluids

كتاب تشريح كامل مسائل مقدمه اي بر مكانيك محيط هاي پيوسته، مشتمل بر 246 صفحه، با فرمت PDF، به زبان انگليسي، كليه مسائل كتاب بالا (Introduction to Continuum Mechanics) را بطور كامل تشريح نموده است.

جهت دانلود مجموعه آموزشي مكانيك محيط هاي پيوسته به همراه تشريح كامل مسائل، برلينك زير كليك نماييد.

مقدمه اي بر مكانيك محيط هاي پيوسته به همراه تشريح كامل مسائل

اين كتاب درسي به صورت متعادل و با استفاده از ترموديناميك، رفتار جامدات و مايعات را بررسي مي نمايد. در اين كتاب همچنين مطالب رياضيات پيشرفته جهت فهم بهتر فصل هاي اوليه كتاب ارائه شده است. اين كتاب مشتمل بر 150 تمرين و همچنين حاوي مثالهاي كاربردي زيادي مي باشد...

كتاب مقدمه اي بر مكانيك محيط هاي پيوسته (Introduction to Continuum Mechanics)، مشتمل بر 252 صفحه، در 14 فصل، با فرمت PDF، به زبان انگليسي، همراه با مثال ها و تمرينات متعدد به ترتيب زير گردآوري شده است:

Chapter 1: Introduction

• Concept of a Continuum
• Sequence of Topics

Chapter 2: Cartesian Tensors

• Index Notation and Summation Convention
• Kronecker Delta and Permutation Symbol
• Example: Skew Symmetry
• Example: Products
• Coordinate System
• Coordinate Transformations
• Vectors
• Tensors
• Examples of Tensors
• Quotient Rule
• Inner Products: Notation
• Quadratic Forms and Eigenvalue Problems
• Example: Eigenvalue Problem
• Diagonalization and Polar Decomposition
• Example: Polar Decomposition

Chapter 3: General Tensors

• Vectors and Tensors
• Physical Components
• Tensor Calculus
• Curvature Tensors
• Applications
• Example: Incompressible Flow
• Example:Equilibrium of Stresses

Chapter 4: Integral Theorems

• Gauss Theorem
• Stokes Theorem

Chapter 5: Deformation

• Lagrangian and Eulerian Descriptions
• Deformation Gradients
• Deformation Gradient Vectors
• Curvilinear Systems
• Strain Tensors
• Decomposition of Displacement Gradients
• Stretch
• Extension
• Infinitesimal Strains and Rotations
• Deformation Ellipsoids
• Polar Decomposition of the Deformation Gradient
• Stretch and Rotation
• Example: Polar Decomposition
• Example: Square Root of a Matrix
• Logarithmic Strain
• Change of Volume
• Change of Area
• Compatibility Equations
• Spatial Rotation and Two-Point Tensors
• Curvilinear Coordinates

Chapter 6: Motion

• Material Derivative
• Some Terminology
• Example: Path Line, Stream Line, and Streak Line
• Length, Volume, and Area Elements
• Length
• Volume
• Area
• Material Derivatives of Integrals
• Line Integrals
• Area Integrals
• Volume Integrals
• Deformation Rate, Spin, and Vorticity
• Strain Rate
• Rotation Rate of Principal Axis

Chapter 7: Fundamental Laws of Mechanics

• Mass
• Conservation and Balance Laws
• Conservation of Mass
• Balance of Linear Momentum
• Balance of Angular Momentum
• Balance of Energy
• Entropy Production
• Axiom of Material Frame Indifference
• Objective Measures of Rotation
• Integrity Basis

Chapter 8: Stress Tensor

• External Forces and Moments
• Internal Forces and Moments
• Cauchy Stress and Couple Stress Tensors
• Transformation of the Stress Tensor
• Principal Stresses
• Shear Stress
• Hydrostatic Pressure and Deviatoric Stresses
• Objective Stress Rates
• Local Conservation and Balance Laws
• Conservation of Mass
• Balance of Linear Momentum
• Balance of Moment of Momentum (Angular Momentum)
• Material Description of the Equations of Motion
• First Piola–******chhoff Stress Tensor
• Second Piola–******chhoff Stress Tensor

Chapter 9: Energy and Entropy Constraints

• Classical Thermodynamics
• Balance of Energy
• Clausius–Duhem Inequality
• Fourier’s Law of Heat Conduction
• Newton’s Law of Visغير مجاز مي باشدity
• Onsager’s Principle
• Strain Energy Density
• Ideal Gas
• Internal Energy
• Legendre or Contact Transformation
• Surface Energy
• Method of Jacobians in Thermodynamics

Chapter 10: Constitutive Relations

• Invariance Principles
• Principles of Exclusion
• Principle of Coordinate Invariance
• Principle of Spatial Invariance
• Principle of Material Invariance
• Principle of Dimensional Invariance
• Principle of Consistency
• Simple Materials
• Elastic Materials
• Elastic Materials of Cauchy
• Elastic Materials of Green
• Stokes Fluids
• Invariant Surface Integrals
• Singularities

Chapter 11: Hyperelastic Materials

• Finite Elasticity
• Homogeneous Deformation
• Simple Extension
• Hydrostatic Pressure
• Simple Shear
• Torsion of a Circular Cylinder
• Approximate Strain Energy Functions
• Hookean Materials
• Small-Strain Approximation
• Plane Stress and Plane Strain
• Integrated Elasticity
• Example: Incremental Loading
• A Variational Principle for Static Elasticity
• Isotropic Thermoelasticity
• Specific Heats and Latent Heats
• Strain Cooling
• Adiabatic and Isothermal Elastic Modulus
• Example: Rubber Elasticity
• Linear Anisotropic Materials
• Invariant Integrals

Chapter 12: Fluid Dynamics

• Basic Equations
• Approximate Constitutive Relations
• Newtonian Fluids
• Inviscid Fluids
• Shearing Flow
• Pipe Flow
• Rotating Flow
• Navier–Stokes Equations
• Incompressible Flow
• Compressible Flow
• Inviscid Flow
• Speed of Sound
• Method of Characteristics
• Bernoulli Equation
• Invariant Integrals

Chapter 13: Viscoelasticity

• Kelvin–Voigt Solid
• Maxwell Fluid
• Standard Linear Solid
• Superposition Principle
• Constitutive Laws in the Operator Form
• Three-Dimensional Linear Constitutive Relations
• Anisotropy
• Biot’s Theory
• Minimum Entropy Production Rate
• Creep in Metals
• Nonlinear Theories of Viscoelasticity
• K-BKZ Model for Viscoelastic Fluids

Chapter 14: Plasticity

• Idealized Theories
• Rigid Perfectly Plastic Material
• Elastic Perfectly Plastic Material
• Elastic Linearly Hardening Material
• Three-Dimensional Theories
• Postyield Behavior
• Levy–Mises Flow Rule
• Prandtl–Reuss Flow Rule
• General Yield Condition and Plastic Work
• Plane Stress and Plane Strain
• Rigid Plasticity and Slip-Line Field
• Example: Symmetric External Cracks
• Drucker’s Definition of Stability
• Il´ıushin’s Postulate
• Work-Hardening Rules
• Perfectly Plastic Material
• Isotropic Hardening
• Kinematic Hardening
• Hencky’s Deformation Theory
• Endochronic Theory of Valanis
• Plasticity and Damage
• Minimum Dissipation Rate Principle

جهت دانلود كتاب مقدمه اي بر مكانيك محيط هاي پيوسته (Introduction to Continuum Mechanics)، برلينك زير كليك نماييد.

مقدمه اي بر مكانيك محيط هاي پيوسته

پروفسور جي ان ردي يك استاد برجسته در دانشكده مهندسي مكانيك دانشگاه تگزاس مي باشد. اين استاد برجسته در سطح بين المللي به خاطر تبحر در علوم مكانيك محاسباتي و مكانيك كاربردي و همچنين تاليف 17 كتاب و ارائه بيش از 450 مقاله در ژورنال هاي مختلف علمي شناخته شده مي باشد. كتاب مقدمه اي بر مكانيك محيط هاي پيوسته پروفسور ردي فرم غيرمادي و جزء را معرفي مي نمايد. در اين كتاب فرم معادلات اساسي و برنامه هاي كاربردي آنها به مشكلات در كشش، مكانيك سيالات، و انتقال حرارت و معرفي مختصر به ويسكوالاستيك خطي را نيز ارائه مي دهد. اين كتاب براي دانشجويان تحصيلات تكميلي بسيار ايده آل مي باشد. همچنين اين كتاب براي كساني كه به دنبال كار بيشتر در زمينه هاي ديناميك سيالات، انعطاف پذيري، صفحات و پوسته ها، ويسكوزولاسيون، پلاستيك و زمينه هاي بين رشته اي مانند ژئومكانيك، بيومكانيك، مكانيولوژي و علوم نانو هستند مي تواند مفيد واقع گردد...

كتاب مقدمه اي بر مكانيك محيط هاي پيوسته (Introduction to Continuum Mechanics)، مشتمل بر 480 صفحه، در 9 فصل، با فرمت PDF، به زبان انگليسي، همراه با مثال ها و تمرينات متعدد به ترتيب زير گردآوري شده است:

Chapter 1: Introduction

• Continuum Mechanics
• A Look Forward
• Summary
• Problems

Chapter 2: VECTORS AND TENSORS

• Background and Overview
• Vector Algebra
• Definition of a Vector
• Vector addition
• Multiplication of a vector by a scalar
• Linear independence of vectors
• Scalar and Vector Products
• Scalar product
• Vector product
• Triple products of vectors
• Plane Area as a Vector
• Reciprocal Basis
• Components of a vector
• General basis
• Ortho normal basis
• The Gram–Schmidt ortho normalization
• Summation Convention
• Dummy index
• Free index
• Kronecker delta
• Permutation symbol
• Transformation Law for Different Bases
• General transformation laws
• Transformation laws for orthonormal systems
• Theory of Matrices
• Definition
• Matrix Addition and Multiplication of a Matrix by a Scalar
• Matrix Transpose
• Symmetric and Skew Symmetric Matrices
• Matrix Multiplication
• Inverse and Determinant of a Matrix
• Positive-Definite and Orthogonal Matrices
• Vector Calculus
• Differentiation of a Vector with Respect to a Scalar
• .Curvilinear Coordinates
• The Fundamental Metric
• Derivative of a Scalar Function of aVector
• The Del Operator
• Divergence and Curl of a Vector
• Cylindrical and Spherical Coordinate Systems
• Gradient, Divergence, and Curl Theorems
• Tensors
• Dyads and Dyadics
• Nonion Form of a Second-Order Tensor
• Transformation of Components of a Tensor
• Higher-Order Tensors
• Tensor Calculus
• Eigenvalues and Eigenvectors
• Eigenvalue problem
• Eigenvalues and eigen vectors of a real symmetric tensor
• Spectral theorem
• Calculation of eigenvalues and eigen vectors
• Summary
• Problems

Chapter 3: KINEMATICS OF CONTINUA

• Introduction
• Descriptions of Motion
• Configurations of a Continuous Medium
• Material Description
• Spatial Description
• Displacement Field
• Analysis of Deformation
• Deformation Gradient
• Isochoric, Homogeneous, and In homogeneous Deformation
• Isochoric deformation
• Homogeneous deformation
• Nonhomogeneous deformation
• Change of Volume and Surface
• Volume change
• Area change
• Strain Measures
• Cauchy Green Deformation Tensors
• Green Lagrange Strain Tensor
• Physical Interpretation of Green–Lagrange Strain Components
• Cauchy and Euler Strain Tensors
• Transformation of Strain Components
• Invariants and Principal Values of Strains
• Infinitesimal Strain Tensor and Rotation Tensor
• Infinitesimal Strain Tensor
• Physical Interpretation of Infinitesimal Strain
• Tensor Components
• Infinitesimal Rotation Tensor
• Infinitesimal Strains in Cylindrical and Spherical
• Coordinate Systems
• Cylindrical coordinate system
• Spherical coordinate system
• Velocity Gradient and Vorticity Tensors
• Relationship Between D and ˙E
• Compatibility Equations
• Preliminary Comments
• Infinitesimal Strains
• Finite Strains
• Rigid-Body Motions and Material Objectivity
• Superposed Rigid-Body Motions
• Introduction and rigid-body transformation
• Effect on F
• Effect on C and E
• Effect on L and D
• Material Objectivity
• Observer transformation
• Objectivity of various kinematic measures
• Time rate of change in a rotating frame of reference
• Polar Decomposition Theorem
• Preliminary Comments
• Rotation and Stretch Tensors
• Objectivity of Stretch Tensors
• Summary
• Problems

Chapter 4: STRESS MEASURES

• Introduction
• Cauchy Stress Tensor and Cauchy’s Formula
• Stress Vector
• Cauchy’s Formula
• Cauchy Stress Tensor
• Transformation of Stress Components and Principal Stresses
• Transformation of Stress Components
• Invariants
• Transformation equations
• Principal Stresses and Principal Planes
• Maximum Shear Stress
• Other Stress Measures
• Preliminary Comments
• First Piola ******chhoff Stress Tensor
• Second Piola ******chhoff Stress Tensor
• Equilibrium Equations for Small Deformations
• Objectivity of Stress Tensors
• Cauchy Stress Tensor
• First Piola ******chhoff Stress Tensor
• Second Piola ******chhoff Stress Tensor
• Summary
• Problems

Chapter 5: CONSERVATION AND BALANCE LAWS

• Introduction
• Conservation of Mass
• Preliminary Discussion
• Material Time Derivative
• Vector and Integral Identities
• Vector identities
• Integral identities
• Continuity Equation in the Spatial Description
• Continuity Equation in the Material Description
• Reynolds Transport Theorem
• Balance of Linear and Angular Momentum
• Principle of Balance of Linear Momentum
• Equations of motion in the spatial description
• Equations of motion in the material description
• Spatial Equations of Motion in Cylindrical and Spherical Coordinates
• Cylindrical coordinates
• Spherical coordinates
• Principle of Balance of Angular Momentum
• Mono polar case
• Multi polar case
• Thermodynamic Principles
• Balance of Energy
• Energy equation in the spatial description
• Energy equation in the material description
• Entropy Inequality
• Homogeneous processes
• In homogeneous processes
• Conservation and Balance Equations in the Spatial Description
• Conservation and Balance Equations in the Material Description
• Closing Comments
• Problems

Chapter 6: CONSTITUTIVE EQUATIONS

• Introduction
• General Comments
• General Principles of Constitutive Theory
• Material Frame Indifference
• Restrictions Placed by the Entropy Inequality
• Elastic Materials
• Cauchy Elastic Materials
• Green-Elastic or Hyper elastic Materials
• Linearized Hyper elastic Materials: Infinitesimal Strains
• Hookean Solids
• Generalized Hooke’s Law
• Material Symmetry Planes
• Monoclinic Materials
• Orthotropic Materials
• Isotropic Materials
• Nonlinear Elastic Constitutive Relations
• Newtonian Fluids
• Ideal Fluids
• Viscous In compressible Fluids
• Generalized Newtonian Fluids
• Inelastic Fluids
• Power law model
• Carreau model
• Bingham model
• Visco elastic Constitutive Models
• Differential models
• Integral models
• Heat Transfer
• Fourier’s Heat Conduction Law
• Newton’s Law of Cooling
• Stefan–Boltzmann Law
• Constitutive Relations for Coupled Problems
• Electro magnetics
• Maxwell’s equations
• Constitutive relations
• Thermo elasticity
• Hygro thermal elasticity
• Electro elasticity
• Summary
• Problems

Chapter 7: LINEARIZED ELASTICITY

• Introduction
• Governing Equations
• Preliminary Comments
• Summary of Equations
• Strain displacement equations
• Equations of motion
• Constitutive equations
• Boundary conditions
• Compatibility conditions
• The Navier Equations
• The Beltrami Michell Equations
• Solution Methods
• Types of Problems
• Types of Solution Methods
• Examples of the Semi Inverse Method
• Stretching and Bending of Beams
• Superposition Principle
• Uniqueness of Solutions
• Clapeyron’s, Betti’s, and Maxwell’s Theorems
• Clapeyron’s Theorem
• Betti’s Reciprocity Theorem
• Maxwell’s Reciprocity Theorem
• Solution of Two-Dimensional Problems
• Plane Strain Problems
• Plane Stress Problems
• Unification of Plane Stress and Plane Strain Problems
• Airy Stress Function
• Saint Venant’s Principle
• Torsion of Cylindrical Members
• Warping function
• Prandtl’s stress function
• Methods Based on Total Potential Energy
• The Variational Operator
• The Principle of the Minimum Total Potential Energy
• Construction of the total potential energy functional
• Euler’s equations and natural boundary conditions
• Minimum property of the total potential energy functional
• Castigliano’s TheoremI
• The Ritz Method
• The variational problem
• Description of the method
• Hamilton’s Principle
• Hamilton’s Principle for a Rigid Body
• Hamilton’s Principle for a Continuum
• Summary
• Problems

Chapter 8: FLUID MECHANICS AND HEAT TRANSFER

• Governing Equations
• Preliminary Comments
• Summary of Equations
• Fluid Mechanics Problems
• Governing Equations of Viscous Fluids
• In viscid Fluid Statics
• Parallel Flow (Navier Stokes Equations)
• Problems with Negligible Convective Terms
• Energy Equation for One-Dimensional Flows
• Heat Transfer Problems
• Governing Equations
• Heat Conduction in a Cooling Fin
• Axisymmetric Heat Conduction in a Circular Cylinder
• Two Dimensional Heat Transfer
• Coupled Fluid Flow and Heat Transfer
• Summary
• Problems

Chapter 9: LINEARIZED VISCOELASTICITY

• Introduction
• Preliminary Comments
• Initial Value Problem, the Unit Impulse, and the Unit Step Function
• The Laplace Transform Method
• Spring and Dashpot Models
• Creep Compliance and Relaxation Modulus
• Maxwell Element
• Creep response
• Relaxation response
• Kelvin Voigt Element
• Creep response
• Relaxation response
• Three Element Models
• Four Element Models
• Integral Constitutive Equations
• Hereditary Integrals
• Hereditary Integrals for Deviatoric Components
• The Correspondence Principle
• Elastic and Viscoelastic Analogies
• Summary
• Problems

جهت دانلود كتاب مقدمه اي بر مكانيك محيط هاي پيوسته (Introduction to Continuum Mechanics)، برلينك زير كليك نماييد.

مقدمه اي بر مكانيك محيط هاي پيوسته